Portal:Algebra
2008/9 Schools Wikipedia Selection. Related subjects: Mathematics; Portals
Algebra is a branch of mathematics concerning the study of structure, relation and quantity. The name is derived from the treatise written by the muslim Persian mathematician, astronomer, astrologer and geographer, Muhammad bin Mūsā al-Khwārizmī titled Kitab al-Jabr wa-l-Muqabala (meaning " The Compendious Book on Calculation by Completion and Balancing"), which provided symbolic operations for the systematic solution of linear and quadratic equations.
Together with geometry, analysis, combinatorics, and number theory, algebra is one of the main branches of mathematics. Elementary algebra is often part of the curriculum in secondary education and provides an introduction to the basic ideas of algebra, including effects of adding and multiplying numbers, the concept of variables, definition of polynomials, along with factorization and determining their roots.
Algebra is much broader than elementary algebra and can be generalized. In addition to working directly with numbers, algebra covers working with symbols, variables, and set elements. Addition and multiplication are viewed as general operations, and their precise definitions lead to structures such as groups, rings and fields.
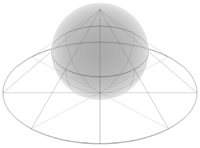
| 3D illustration of a stereographic projection from the north pole onto a plane below the sphere. |
The stereographic projection is a particular mapping (function) that projects a sphere onto a plane. The projection is defined on the entire sphere, except at one point — the projection point. Where it is defined, the mapping is smooth and bijective. It is also conformal, meaning that it preserves angles. On the other hand, it does not preserve area, especially near the projection point.
Intuitively, then, the stereographic projection is a way of picturing the sphere as the plane, with some inevitable compromises. Because the sphere and the plane appear in many areas of mathematics and its applications, so does the stereographic projection; it finds use in diverse fields including differential geometry, complex analysis, cartography, geology, and crystallography.
| Image credit: Mark Howison |
- ...that it is impossible to devise a single formula involving only polynomials and radicals for solving an arbitrary quintic equation?
- ...that it is possible for a three dimensional figure to have a finite volume but infinite surface area? An example of this is Gabriel's Horn.
- ...that the Gudermannian function relates the regular trigonometric functions and the hyperbolic trigonometric functions without the use of complex numbers?
- ...that the classification of finite simple groups was not completed until the mid 1980s?
- ...that a field is an algebraic structure in which the operations of addition, subtraction, multiplication and division (except division by zero) may be performed, and the same rules hold which are familiar from the arithmetic of ordinary numbers?