Climate model
2008/9 Schools Wikipedia Selection. Related subjects: Climate and the Weather
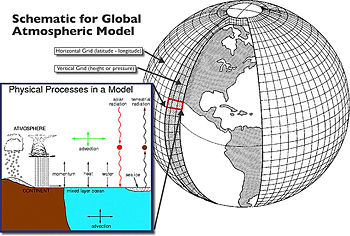
Climate models use quantitative methods to simulate the interactions of the atmosphere, oceans, land surface, and ice. They are used for a variety of purposes from study of the dynamics of the weather and climate system to projections of future climate.
All climate models balance, or very nearly balance, incoming energy as short wave electromagnetic radiation (which in this context means visible and ultraviolet, not to be confused with shortwave) to the earth with outgoing energy as long wave ( infrared) electromagnetic radiation from the earth. Any imbalance results in a change in the average temperature of the earth.
The most talked-about models of recent years have been those relating temperature to emissions of carbon dioxide (see greenhouse gas). These models project an upward trend in the surface temperature record, as well as a more rapid increase in temperature at higher altitudes.
Models can range from relatively simple to quite complex:
- A simple radiant heat transfer model that treats the earth as a single point and averages outgoing energy
- this can be expanded vertically (radiative-convective models), or horizontally
- finally, (coupled) atmosphere–ocean–sea ice global climate models discretise and solve the full equations for mass and energy transfer and radiant exchange.
This is not a full list; for example "box models" can be written to treat flows across and within ocean basins.
Zero-dimensional models
A very simple model of the radiative equilibrium of the Earth is
- (1 − a)Sπr2 = 4πr2εσT4
where
- the left hand side represents the incoming energy from the Sun
- the right hand side represents the outgoing energy from the Earth, calculated from the Stefan-Boltzmann law assuming a constant radiative temperature, T, that is to be found,
and
- S is the solar constant - the incoming solar radiation per unit area - about 1367 W·m-2
- a is the Earth's average albedo, measured to be 0.3
- r is Earth's radius — approximately 6.371×106m
- π is well known, approximately 3.14159
- σ is the Stefan-Boltzmann constant — approximately 5.67×10-8 J·K-4·m-2·s-1
- ε is the effective emissivity of earth, about 0.612
The constant πr2 can be factored out, giving
- (1 − a)S = 4εσT4
This yields an average earth temperature of 288 K . This is because the above equation represents the effective radiative temperature of the Earth (including the clouds and atmosphere). The use of effective emissivity accounts for the greenhouse effect.
This very simple model is quite instructive, and the only model that could fit on a page. For example, it easily determines the effect on average earth temperature of changes in solar constant or change of albedo or effective earth emissivity. Using the simple formula, the percent change of the average amount of each parameter, considered independently, to cause a one degree Celsius change in steady-state average earth temperature is as follows:
Solar constant 1.4% Albedo 3.3% Effective emissivity 1.4%
The average emissivity of the earth is readily estimated from available data. The emissivities of terrestrial surfaces are all in the range of 0.96 to 0.99 (except for some small desert areas which may be as low as 0.7). Clouds, however, which cover about half of the earth’s surface, have an average emissivity of about 0.5 (which must be reduced by the fourth power of the ratio of cloud absolute temperature to average earth absolute temperature) and an average cloud temperature of about 258 K . Taking all this properly into account results in an effective earth emissivity of about 0.64 (earth average temperature 285 K).
This simple model readily determines the effect of changes in solar output or change of earth albedo or effective earth emissivity on average earth temperature. It says nothing, however about what might cause these things to change. Zero-dimensional models do not address the temperature distribution on the earth or the factors that move energy about the earth.
Radiative-Convective Models
The zero-dimensional model above, using the solar constant and given average earth temperature, determines the effective earth emissivity of long wave radiation emitted to space. This can be refined in the vertical to a zero-dimensional radiative-convective model, which considers two processes of energy transport:
- upwelling and downwelling radiative transfer through atmospheric layers that both absorb and emit infrared radiation
- upward transport of heat by convection (especially important in the lower troposphere).
The radiative-convective models have advantages over the simple model: they can determine the effects of varying greenhouse gas concentrations on effective emissivity and therefore the surface temperature. But added parameters are needed to determine local emissivity and albedo and address the factors that move energy about the earth.
Links:
- http://www.giss.nasa.gov/gpol/abstracts/1980/WangStone.html
- http://www.grida.no/climate/ipcc_tar/wg1/258.htm
Higher Dimension Models
The zero-dimensional model may be expanded to consider the energy transported horizontally in the atmosphere. This kind of model may well be zonally averaged. This model has the advantage of allowing a rational dependence of local albedo and emissivity on temperature - the poles can be allowed to be icy and the equator warm - but the lack of true dynamics means that horizontal transports have to be specified.
- http://www.shodor.org/master/environmental/general/energy/application.html
GCMs (Global Climate Models or General circulation models)
Three (or more properly, four since time is also considered) dimensional GCM's discretise the equations for fluid motion and energy transfer and integrate these forward in time. They also contain parametrisations for processes - such as convection - that occur on scales too small to be resolved directly.
Atmospheric GCMs (AGCMs) model the atmosphere and impose sea surface temperatures. Coupled atmosphere-ocean GCMs (AOGCMs, e.g. HadCM3, EdGCM, GFDL CM2.X, ARPEGE-Climat ) combine the two models. The first general circulation climate model that combined both oceanic and atmospheric processes was developed in the late 1960s at the NOAA Geophysical Fluid Dynamics Laboratory AOGCMs represent the pinnacle of complexity in climate models and internalise as many processes as possible. However, they are still under development and uncertainties remain.
Most recent simulations show "plausible" agreement with the measured temperature anomalies over the past 150 years, when forced by observed changes in "Greenhouse" gases and aerosols, but better agreement is achieved when natural forcings are also included .
Climate models on the web
- Dapper/DChart - plot and download model data referenced by the Fourth Asssessment Report (AR4) of the Intergovernmental Panel on Climate Change .
- http://www.hadleycentre.gov.uk/research/hadleycentre/models/modeltypes.html - Hadley Centre for Climate Prediction and Research - general info on their models
- http://www.ccsm.ucar.edu/ - NCAR/ UCAR Community Climate System Model (CCSM)
- http://www.climateprediction.net - do it yourself climate prediction
- http://edgcm.columbia.edu/ - a NASA/GISS global climate model (GCM) with a user-friendly interface for PCs and Macs
- http://www.cccma.bc.ec.gc.ca/ - CCCma model info and interface to retrieve model data
- http://nomads.gfdl.noaa.gov/CM2.X/ - NOAA / Geophysical Fluid Dynamics Laboratory CM2 global climate model info and model output data files
- http://www.climate.uvic.ca/ - University of Victoria Global climate model, free for download. Leading researcher was a contributing author to the recent IPCC report on climate change.