Octahedron

2008/9 Schools Wikipedia Selection. Related subjects: Mathematics

Regular Octahedron
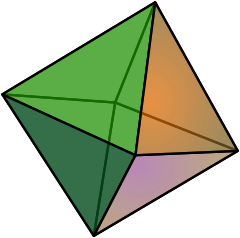(Click here for rotating model)
Type	Platonic solid
Elements	F = 8, E = 12 V = 6 (χ = 2)
Faces by sides	8{3}
Schläfli symbol	{3,4} and t₁{3,3}
Wythoff symbol	4 \| 2 3 2 \| 3 3 \| 3 2 2
Coxeter-Dynkin
Symmetry	O_h
References	U₀₅, C₁₇, W₂
Properties	Regular convex deltahedron
Dihedral angle	109.47122° = arccos(-1/3)
3.3.3.3 ( Vertex figure)	Cube ( dual polyhedron)
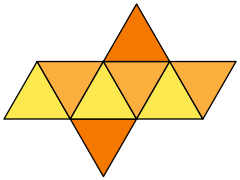Net

An octahedron (plural: octahedra) is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight equilateral triangles, four of which meet at each vertex.

The octahedron's symmetry group is O_h, of order 48. This group's subgroups include D_3d (order 12), the symmetry group of a triangular antiprism; D_4h (order 16), the symmetry group of a square bipyramid; and T_d (order 24), the symmetry group of a rectified tetrahedron. These symmetries can be emphasized by different decorations of the faces.

It is a three-dimensional cross polytope.

Cartesian coordinates

An octahedron can be placed with its centre at the origin and its vertices on the coordinate axes; the Cartesian coordinates of the vertices are then

( ±1, 0, 0 );

( 0, ±1, 0 );

( 0, 0, ±1 ).

Area and volume

The area A and the volume V of a regular octahedron of edge length a are:

$A=2\sqrt{3}a^2 \approx 3.46410162a^2$

$V=\frac{1}{3} \sqrt{2}a^3 \approx 0.471404521a^3$

Thus the volume is four times that of a regular tetrahedron with the same edge length, while the surface area is twice (because we have 8 vs. 4 triangles).

Geometric relations

The interior of the compound of two dual tetrahedra is an octahedron, and this compound, called the stella octangula, is its first and only stellation. Correspondingly, a regular octahedron is the result of cutting off from a regular tetrahedron, four regular tetrahedra of half the linear size (i.e. rectifying the tetrahedron). The vertices of the octahedron lie at the midpoints of the edges of the tetrahedron, and in this sense it relates to the tetrahedron in the same way that the cuboctahedron and icosidodecahedron relate to the other Platonic solids. One can also divide the edges of an octahedron in the ratio of the golden mean to define the vertices of an icosahedron. This is done by first placing vectors along the octahedron's edges such that each face is bounded by a cycle, then similarly partitioning each edge into the golden mean along the direction of its vector. There are five octahedra that define any given icosahedron in this fashion, and together they define a regular compound.

Octahedra and tetrahedra can be alternated to form a vertex, edge, and face-uniform tessellation of space, called the octet truss by Buckminster Fuller. This is the only such tiling save the regular tessellation of cubes, and is one of the 28 convex uniform honeycombs. Another is a tessellation of octahedra and cuboctahedra.

The octahedron is unique among the Platonic solids in having an even number of faces meeting at each vertex. Consequently, it is the only member of that group to possess mirror planes that do not pass through any of the faces.

Using the standard nomenclature for Johnson solids, an octahedron would be called a square bipyramid.

Related polyhedra

The octahedron can also be considered a rectified tetrahedron. This can be shown by a 2-colour face model. With this coloring, the octahedron has tetrahedral symmetry.

Compare this truncation sequence between a tetrahedron and its dual:

Tetrahedron

Truncated tetrahedron

octahedron

Truncated tetrahedron

Tetrahedron

Octahedra in the physical world

Fluorite octahedron.

Especially in roleplaying games, this solid is known as a d8, one of the more common Polyhedral dice.

If each edge of an octahedron is replaced by a one ohm resistor, the resistance between opposite vertices is 1/2 ohms, and that between adjacent vertices 5/12 ohms.

Natural crystals of diamond, alum or fluorite are commonly octahedral.

Octahedra in music

If you place notes on every vertex of an octahedron, you can get a six note just intonation scale with remarkable properties - it is highly symmetrical and has eight consonant triads and twelve consonant diads. See hexany

Other octahedra

The regular octahedron has 6 vertices and 12 edges, the minimum for an octahedron; nonregular octahedra may have as many as 12 vertices and 18 edges.

Hexagonal prism: 6 squares, 2 hexagons
Heptagonal pyramid: 7 triangles, 1 heptagon
Tetragonal bipyramid: 8 triangles, usually isosceles)
- The regular octahedron is a special case with equilateral triangles
Truncated tetrahedron: 4 triangles, 4 hexagons
Tetragonal trapezohedron - 8 kites

Retrieved from " http://en.wikipedia.org/wiki/Octahedron"