Image:Close-packed spheres.jpg

From Wikipedia, the free encyclopedia

Summary

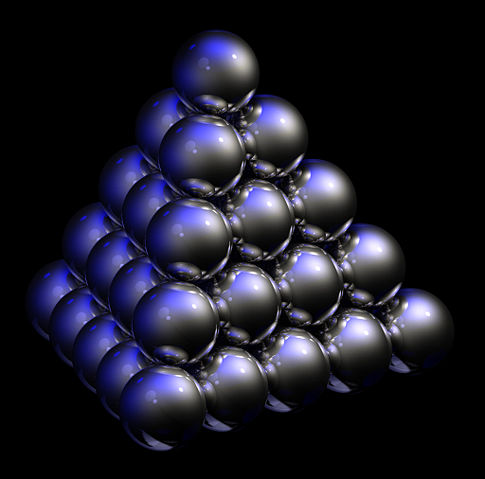
Shown above is what the science of sphere packing calls a closest-packed arrangement. Specifically, this is the cannonball arrangement or cannonball stack. Thomas Harriot in ca. 1585 first pondered the mathematics of cannonball stacks and later asked Johannes Kepler if the stack illustrated here was truly the most efficient. Kepler wrote, in what today is known as the Kepler conjecture, that no other arrangement of spheres can exceed its packing density of 74%.

Mathematically, there is an infinite quantity of closest-packed arrangements (assuming an infinite-size volume in which to arrange spheres). In the field of crystal structure however, unit cells (a crystal’s repeating pattern) are composed of a limited number of atoms and this reduces the variety of closest-packed regular lattices found in nature to only two: hexagonal close packed (HCP), and face-centered cubic (FCC). As can be seen at this site at King’s College, there is a distinct, real difference between different lattices; it’s not just a matter of how one slices 3D space. With all closest-packed lattices however, any given internal atom is in contact with 12 neighbors — the maximum possible.

Note that this stack is not an FCC unit cell since this group can not tessellate in 3D space. Visit the King’s College Web site to see HCP and FCC unit cells.

When many chemical elements (such as most of the noble gases and platinum-group metals) freeze solid, their lattice unit cells are of the FCC form. Having a closest-packed arrangement is one of the reasons why iridium and osmium (both of which are platinum-group metals) have the two greatest bulk densities of all the chemical elements.

The stack shown here is indeed quite dense. If this stack of 35 spheres was composed of iron cannonballs, each measuring 10 cm in diameter, the top of the stack would be only 42.66 cm off the ground — just under the knee of the average barefoot man — and yet would weigh over 144 kg.


  1. ^ To 23 significant digits, the value is 74.048 048 969 306 104 116 931%


Rendered and modeled using Ashlar Incorporated’s Cobalt on a Mac.

Licensing

GFDL I, the creator of this work, hereby grant the permission to copy, distribute and/or modify this document under the terms of the GNU Free Documentation License, Version 1.2 or any later version published by the Free Software Foundation; with no Invariant Sections, no Front-Cover Texts, and no Back-Cover Texts. Subject to disclaimers.
This file is an exact duplicate of another file from the Wikimedia Commons. Unless it is currently protected from editing, this media file may qualify for speedy deletion if it satisfies these conditions.

File history

Click on a date/time to view the file as it appeared at that time.

Date/Time Dimensions User Comment
current 17:04, 18 February 2007 1,024×1,012 (246 KB) Greg L ( Talk | contribs) (Shown above is what the science of sphere packing calls a '''''cubic closest-packed arrangement.''''' Per the Kepler conjecture, this arrangement of spheres (also known as the ''cannonball arrangement)'' has the greatest packing density of any o)
revert 16:53, 18 February 2007 1,024×1,012 (252 KB) Greg L ( Talk | contribs) (Shown above is what the science of sphere packing calls a '''''cubic closest-packed arrangement.''''' Per the Kepler conjecture, this arrangement of spheres (also known as the ''cannonball arrangement)'' has the greatest packing density of any o)
revert 07:37, 18 February 2007 1,024×952 (164 KB) Greg L ( Talk | contribs) (Shown above is what in the science of sphere packing, is known as a '''''cubic closest-packed arrangement.''''' Per the Kepler conjecture, this arrangement of spheres (also known as the ''cannonball arrangement)'' has the greatest packing densit)
revert 03:34, 18 February 2007 1,024×1,312 (186 KB) Greg L ( Talk | contribs) (Shown above is what in the science of sphere packing, is known as a '''''cubic closest-packed arrangement.''''' Per the Kepler conjecture, this arrangement of spheres (also known as the ''cannonball arrangement)'' has the greatest packing densit)
revert 19:14, 17 February 2007 1,024×1,024 (159 KB) Greg L ( Talk | contribs) (Shown here is an octahedron of spheres. In the science of sphere packing, this arrangement is a ''closest-packed structure.'' As per the Kepler conjecture, this arrangement of spheres (also known as the ''cannonball arrangement)'' has the gre)

    See the setup instructions for more information.

The following file is a duplicate of this file:

The following pages on Schools Wikipedia link to this image (list may be incomplete):

Metadata

This file contains additional information, probably added from the digital camera or scanner used to create or digitize it. If the file has been modified from its original state, some details may not fully reflect the modified file.

Orientation Normal
Horizontal resolution 72 dpi
Vertical resolution 72 dpi
Software used Adobe Photoshop 7.0
File change date and time 09:01, 18 February 2007
Colour space 65535
Retrieved from "http://en.wikipedia.org/wiki/Image:Close-packed_spheres.jpg"
The Schools Wikipedia was sponsored by a UK Children's Charity, SOS Children UK , and is mainly selected from the English Wikipedia with only minor checks and changes (see www.wikipedia.org for details of authors and sources). See also our Disclaimer.